
Introduction to Polyhedra
The Platonic Solids
There are only five convex polyhedra for which each face is the same regular polygon and all vertices are of the same type. These are known as the Platonic polyhedra, or Platonic solids. These were known before Plato, but he and his students are known to have studied them, and details regarding the original discovery of these polyhedra are not known. The five are:
- Tetrahedron - 4 faces, each an equilateral triangle
- Hexahedron (Cube) - 6 faces, each a square
- Octahedron - 8 faces, each an equilateral triangle
- Dodecahedron - 12 faces, each a regular pentagon
- Icosahedron - 20 faces, each an equilateral triangle
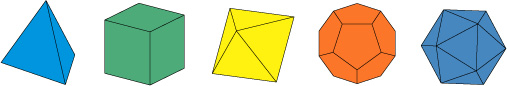
A convex polyhedron is one for which none of the faces form "indentations" on the surface. For the Platonic solids, there is a circumscribing sphere which each of the vertices touches. In addition, the dihedral angle, the angle between two adjacent faces, is the same for any pair of faces for each Platonic solid.
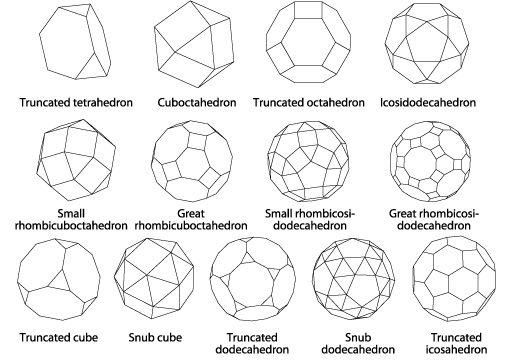
The Archimedean Solids
There are thirteen convex polyhedra for which each face is a regular polygon, with more than one type of regular polygon, and for which all vertices are of the same type. In addition, there are an infinite number of facially regular prisms and antiprisms. These thirteen solids were known to Archimedes, but his writings on them were lost, together with the knowledge of their construction. They were gradually rediscovered during the Renaissance.
 Other Polyhedra
Other Polyhedra
In addition to the above, there are innumerable other polyhedra. There are an infinite number of prisms, antiprisms, and pyramids. There are also concave polyhedra with regular faces, and of course polyhedra with irregular faces. R. Buckminster Fuller used triangles to approximate spheres to create his geodesic polyhedra.
Click here to browse products related to polyhedra.
Click here for unique polyhedral dice.
Math + Art topics
mathartfun.com home
© 2000-2015 Tessellations



